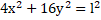A small ball of mass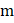 
is attached with upper end of a uniform straight rod of equal mass 
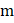and length 
The rod is held vertical over a smooth horizontal surface as shown in fig. When the system is released, the lower end slips freely and the systems falls down. Assuming the initial position of the lower end to be origin and initially rod to be along 
-axis as shown in fig., the equation of trajectory of point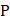 
of the rod (
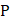is at distance 
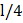from the lower end)is
.png)
|
a) |
|
b) |
|
c) |
|
d) |
|
Question ID - 100141 | SaraNextGen Answer A small ball of mass 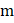is attached with upper end of a uniform straight rod of equal mass 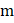and length The rod is held vertical over a smooth horizontal surface as shown in fig. When the system is released, the lower end slips freely and the systems falls down. Assuming the initial position of the lower end to be origin and initially rod to be along -axis as shown in fig., the equation of trajectory of point 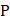of the rod ( 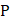is at distance 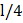from the lower end)is
a)
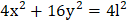
b)
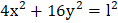
c)
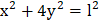
d)
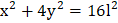
A small ball of mass 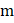is attached with upper end of a uniform straight rod of equal mass 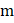and length The rod is held vertical over a smooth horizontal surface as shown in fig. When the system is released, the lower end slips freely and the systems falls down. Assuming the initial position of the lower end to be origin and initially rod to be along -axis as shown in fig., the equation of trajectory of point 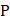of the rod ( 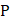is at distance 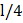from the lower end)is
|
a) |
|
b) |
|
c) |
|
d) |
|
(b)
Since the floor is smooth, therefore no horizontal force is acting on the system. Only three forces are acting on it, weight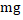 
of the ball, weight 
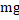of the rod and vertically upward reaction of the floor
.png)
Since the rod is released from rest and no horizontal force is acting on it, therefore centre of mass of the system will not displace horizontally; it means it will fall vertically downward
Since masses of the rod and ball are equal, therefore centre of mass ‘
’ is at mid-point of centre of the rod and the ball; it means at distance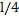 
from the ball or 3/4 from the lower end
If at an instant the rod makes angle 
with the vertical, then point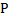 
and the system will be as shown in the figure
Co-ordinates of the point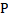 
are
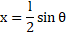
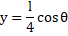
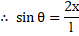
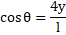
Using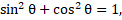 
we have
