A pendulum is executing simple harmonic motion and its maximum kinetic energy is K1. If the length of the pendulum is doubled and it performs simple harmonic motion with the same amplitude as in the first case, its maximum kinetic energy is K2. Then :
|
a. |
K2 = |
b. |
K2 = |
|
c. |
K2 = 2K1 |
d. |
K2 = K1 |
Question ID - 50365 | SaraNextGen Answer A pendulum is executing simple harmonic motion and its maximum kinetic energy is K1. If the length of the pendulum is doubled and it performs simple harmonic motion with the same amplitude as in the first case, its maximum kinetic energy is K2. Then :
a.
K2 =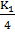
b.
K2 =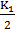
c.
K2 = 2K1
d.
K2 = K1
A pendulum is executing simple harmonic motion and its maximum kinetic energy is K1. If the length of the pendulum is doubled and it performs simple harmonic motion with the same amplitude as in the first case, its maximum kinetic energy is K2. Then :
|
a. |
K2 = |
b. |
K2 = |
|
c. |
K2 = 2K1 |
d. |
K2 = K1 |
1 Answer - 5876 Votes
3537
Answer Key : (c) -
Answer LinkMaximum kinetic energy at lowest point B is given by
K = mg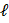
(1 − cos 
)
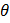where 
= angular amp.
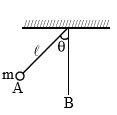
K1 = mg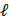
(1 − cos 
)
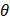K2 = mg(2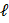
)(1 − cos 
)
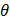K2 = 2K1.
