A ball of mass 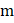 moving with velocity
moving with velocity 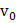 makes an oblique elastic collision with a stationary ball of mass 2
makes an oblique elastic collision with a stationary ball of mass 2 The angle of divergence between the balls after collision in ground frame, if the ball of mass
The angle of divergence between the balls after collision in ground frame, if the ball of mass 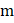 turns by an angle of
turns by an angle of  in centre of mass frame is
in centre of mass frame is
a)

b)

c)
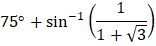
d)
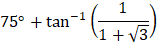
A ball of mass 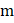 moving with velocity
moving with velocity 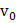 makes an oblique elastic collision with a stationary ball of mass 2
makes an oblique elastic collision with a stationary ball of mass 2 The angle of divergence between the balls after collision in ground frame, if the ball of mass
The angle of divergence between the balls after collision in ground frame, if the ball of mass 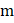 turns by an angle of
turns by an angle of  in centre of mass frame is
in centre of mass frame is
a)

b)

c)
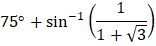
d)
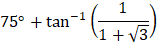
Question ID - 100211 | SaraNextGen Answer
A ball of mass  moving with velocity
moving with velocity  makes an oblique elastic collision with a stationary ball of mass 2
makes an oblique elastic collision with a stationary ball of mass 2 The angle of divergence between the balls after collision in ground frame, if the ball of mass
The angle of divergence between the balls after collision in ground frame, if the ball of mass 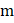 turns by an angle of
turns by an angle of  in centre of mass frame is
in centre of mass frame is
a)

b)

c)
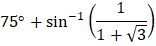
d)
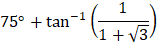
|
A ball of mass |
|||||||
|
a) |
|
b) |
|
c) |
|
d) |
|
(d)
Let us solve this question in  frame. As the collision is elastic, the magnitude of momenta of all balls remains same after collision in this frame
frame. As the collision is elastic, the magnitude of momenta of all balls remains same after collision in this frame
Velocity of  frame w.r.t. ground frame is
frame w.r.t. ground frame is
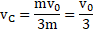
The momentum diagram in  frame is drawn as shown in fig.
frame is drawn as shown in fig.
.png)
Now, velocity of 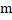 after colision in
after colision in  frame is
frame is 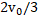 while that of
while that of 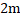 is
is  along
along 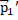 and
and 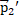 , respectively. Let
, respectively. Let 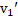 and
and 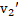 be the velocities of
be the velocities of 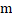 and
and  , respectively after collision in ground frame, then velocity vector diagrams can be drawn as shown
, respectively after collision in ground frame, then velocity vector diagrams can be drawn as shown
.png)
Using trigonometry, from upper triangle
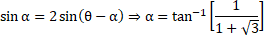
From lower triangle:
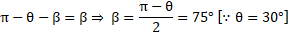
So, angle of divergence is 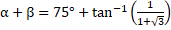
Alternative method Write down conservation of momentum equations, coefficient of restitution equation, energy conservation and use the given conditions
